The meaning of 2-D shapes is 2 dimensional shapes. In Geometry, a two dimensional shape is defined as a plane figure having two dimensions- length, and breadth (or width). 2 dimensional shapes are also known as rectilinear figures or plane figures. Students can also download the HD printout of Area and Perimeter Formulas of all 2 D Shapes and can stick on their pinboard. This will help them to revise and learn all the formulas for doing sums on 2-D shapes.
Area and Perimeter of Plane figures
Before getting into the formulas of area and perimeter of plane figures, let us understand the meaning of Perimeter and Area.
Perimeter: The perimeter of a plane figure is the length of its boundary.
Area: The area of a plane figure is the amount of surface enclosed by its sides.
In simple language, Perimeter is the distance around the outside of a shape and Area is the space inside the shape.
Area and Perimeter Formulas of All 2 D Shapes
Download HD quality printout of Formulas of all 2-D shapes
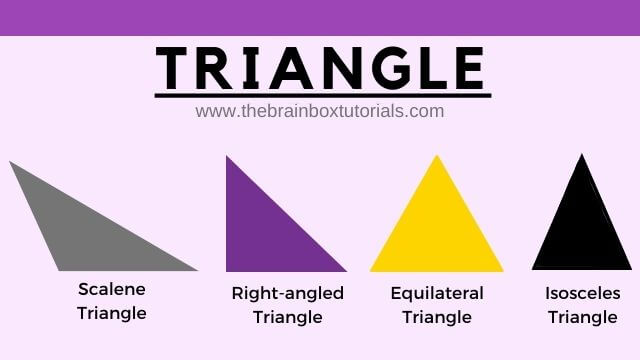
Formulas for Perimeter and Area of Triangle
A Triangle is a three sided closed and plane figure.
- Perimeter of triangle = (a + b + c) units
- Area of triangle (Heron’s formula) = √[s(s – a) (s – b) (s – c)] where s (semi-perimeter) = 1/2 (a + b + c) sq. units
- Area of triangle = 1/2 × base × height sq. units
- Area of an equilateral triangle = (√3a2)/4 sq. units
- Area of an isosceles triangle = b/4 √(4a2– b2) sq. units
- Area of a right-angled triangle = 1/2 × base × height sq. units
Formulas for Perimeter and Area of Parallelogram
A Parallelgram is a four sided closed figure with opposite sides equal and parallel.
- Perimeter of parallelogram = 2 (sum of adjacent sides) units
- Area of parallelogram = (base × correspoding height) sq. units
Formulas for Perimeter and Area of Rhombus
A Rhombus is a four sided closed figure with all sides equal and opposite sides parallel.
- Area of rhombus = (base × corresponding height) sq. units
- Area of rhombus = (1/2 × length of one diagonal × length of other diagonal) sq. units
- Perimeter of rhombus = (4 × side) units
Formulas for Perimeter and Area of Rectangle
If l be the length of the rectangle and b its breadth, then
- Perimeter of rectangle = 2(l + b) units
- Area of rectangle = (l × b) sq. units
- Diagonal of rectangle = √(l² + b²) units
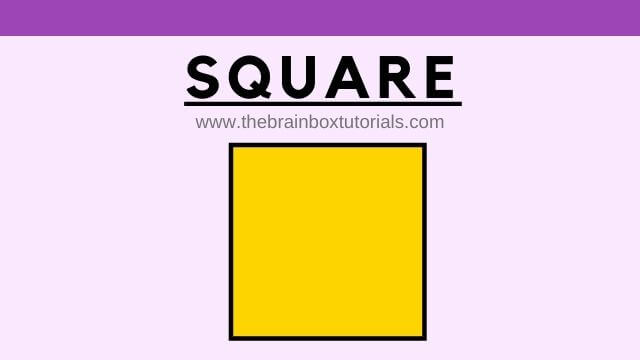
Formulas for Perimeter and Area of Square
- Perimeter of square = 4 a units
- Area of square = a × a = a2 sq. units
- Diagonal of square = (√2)a units
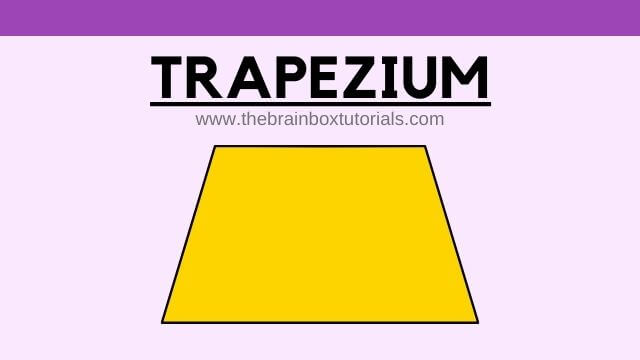
Perimeter and Area of the Trapezium
A Trapezium is a four sided closed figure with any one pair of sides parallel to each other.
- Area of trapezium = 1/2 (sum of parallel sides) × (perpendicular distance between them)
= 1/2 (a + b) × h sq. units
- Perimeter = sum of 4 sides
Circumference and Area of Circle
The path traced by a moving point, which always remains at a fixed distance from a fixed point, is called a Circle.
- Diameter (d) = 2r units
- Circumference of circle = 2πr = πd (where π = 3.14 or 22/7) units
- Area of circle = πr² sq. units
- Area of ring = Area of outer circle – Area of inner circle
= π (R² – r²) sq. units
- 1 revolution by a circular wheel = circumference of that circular wheel
Circumference and Area of Semi-circle
Every diameter divides a circle into two equal parts, each of which is called a Semi-circle.
- Circumference of semi-circle = πr + 2r (where π = 3.14 or 22/7) units
- Area of semi-circle = 1/2 πr² sq. units